Averaging

As a galaxy is never exactly symmetrical there are two methods to obtain a result. In the first method one uses the original image and fits profiles to each quadrant of the galaxy, taking the average of all good profiles. In the second method one averages the galaxy over its four quadrants and only fits the vertical profiles of one quadrant, obtaining the average immediately. For our 1D Disk fit we use the original image fitting the profiles of all four quadrants as it allows us to use the full depth and we can weight the datapoints of each cut appropriately, while using a 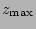$z_\textrm{\scriptsize max}$ as an outer boundary for the vertical height where we think the noise is starting to dominate.
Instead of using the original image like we did for the 1D model and calculating the average afterwards we decided to use a quadrasized average galaxy to obtain an average result immediately as a quadrasized average has several advantages:

1. Intrinsic asymmetric variations in the light distribution are minimized, creating a natural mean image for each galaxy.
2. The S/N ratio is increased as the noise levels are diminished and the intensity of the galaxy becomes more coherent.
3. The flattening of possible asymmetric large scale structures in the background that still remained after the data reduction and the subtraction of the sky image and residual background. As the subtraction of the residual background was done by determining a single mean number of the pixel values around the galaxy there could still be brighter residual structures near the galaxy. This can cause extended vertical structures in the surface brightness profiles which could be mistaken as a hint of a thick disk. Averaging reduces this possible influence.
4. Minimizing the influences of remaining small and faint unmasked stars closely around the galaxy causing individual points in the outer part of the profile to show unwanted excess light. Applying generous mask sizes would mask most of the outer parts of the profile, thus making it impossible to see any hint of a vertical structure.

Following Van der Kruit & Searle vdkruit1981a and Pohlen et. al (2000), we divided the galaxies into their quadrants and averaged the four images. As the masked regions still possess a pixel value that would influence the average, the masked regions were not taken into account. This also removed the chance of having a masked region in our selected profiles. Although using a quadrazised average makes us lose the possibility to study the intrinsic asymmetries of the disk this is not of importance to our research.
To remove the datapoints that clearly belonged to the noise we selected a surface brightness value at which the fainter datapoints would be removed. This cut level was determined by removing all points below the magnitude of the 1, 2 or 3 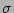$\sigma$ level of the residual background intensity. This cut level depended on the overall quality of each image. Because we wanted to see the faintest parts of the galaxy we went as deep as possible. The corresponding cut level magnitudes, which I will henceforth refer to as 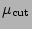$\mu_\textrm{\scriptsize cut}$, have been set to boldface in Table 7 (see columns 4-6).